a b x c d distributive property in boxes Free Distributive Property calculator - Expand using distributive property step-by-step Since CNC machines can process almost any type of material, their applications are near limitless. From direct part production to rapid prototyping, this article looks at the various robust applications of CNC machining.
0 · how to use distributive property
1 · how to solve distributive property
2 · distributive property worksheet
3 · distributive property subtraction
4 · distributive property formula
5 · distributive property for sale
6 · distributive property calculator math
7 · distributive properties of addition
CNC machines offer a high-production output, reduced labor costs, and repeatability. What are the uses of a CNC milling machine? One of the critical benefits of CNC milling is its versatility.
Free Distributive Property calculator - Expand using distributive property step-by-step
The distributive property states that an expression which is given in form of A (B + C) can be solved as A × (B + C) = AB + AC. Understand the distributive property formula along with .
Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without . Distributive Property Calculator is a free online tool that displays the solutions for the given expression using the distributive property. BYJU’S online distributive property calculator tool makes the calculations faster and it . The Distributive Property states that, for real numbers a, b, and c, two conditions are always true: a(b + c) = ab + ac. a(b - c) = ab - ac. You can use distributive property to turn one complex multiplication equation into two . The Distributive Property states that if a, b, c are real numbers, then a(b + c) = ab + ac. In algebra, we use the Distributive Property to remove parentheses as we simplify expressions. When .
how to use distributive property
The distributive property refers to the distributive property of multiplication and applies to both addition and subtraction. An expression in the form A × (B + C) is solved as (A × B) + (A × C) using the distributive property.
The distributive property is the rule that relates addition and multiplication. Specifically, it states that \( a(b+c) = ab + ac \) \( (a+b)c = ac + bc .\) It is a useful tool for expanding expressions, evaluating expressions, and .Learning Target: Apply the Distributive Property to generate equivalent expressions. Success Criteria: • I can explain how to apply the Distributive Property. • I can use the Distributive .
Free Distributive Property calculator - Expand using distributive property step-by-stepThe distributive property states that an expression which is given in form of A (B + C) can be solved as A × (B + C) = AB + AC. Understand the distributive property formula along with examples and FAQs.
how to solve distributive property
Write down the arithmetic equation in the designated box and the tool will simplify it by applying the distribution law, with the steps shown. A distributive property calculator is designed to solve any simple mathematical equation by the basic distribution property. What is Distributive Property In Mathematics?
Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without paper or a calculator by thinking of .97 as 6 − 0.03 and then using the distributive property. Distributive Property Calculator is a free online tool that displays the solutions for the given expression using the distributive property. BYJU’S online distributive property calculator tool makes the calculations faster and it displays the simplification of . The Distributive Property states that, for real numbers a, b, and c, two conditions are always true: a(b + c) = ab + ac. a(b - c) = ab - ac. You can use distributive property to turn one complex multiplication equation into two simpler multiplication problems, then add or subtract the two answers as required. Distributive property of multiplication The Distributive Property states that if a, b, c are real numbers, then a(b + c) = ab + ac. In algebra, we use the Distributive Property to remove parentheses as we simplify expressions. When .
distributive property worksheet
The distributive property refers to the distributive property of multiplication and applies to both addition and subtraction. An expression in the form A × (B + C) is solved as (A × B) + (A × C) using the distributive property. The distributive property is the rule that relates addition and multiplication. Specifically, it states that \( a(b+c) = ab + ac \) \( (a+b)c = ac + bc .\) It is a useful tool for expanding expressions, evaluating expressions, and simplifying expressions.
Learning Target: Apply the Distributive Property to generate equivalent expressions. Success Criteria: • I can explain how to apply the Distributive Property. • I can use the Distributive Property to simplify algebraic expressions.Free Distributive Property calculator - Expand using distributive property step-by-step
The distributive property states that an expression which is given in form of A (B + C) can be solved as A × (B + C) = AB + AC. Understand the distributive property formula along with examples and FAQs.
Write down the arithmetic equation in the designated box and the tool will simplify it by applying the distribution law, with the steps shown. A distributive property calculator is designed to solve any simple mathematical equation by the basic distribution property. What is Distributive Property In Mathematics? Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without paper or a calculator by thinking of .97 as 6 − 0.03 and then using the distributive property. Distributive Property Calculator is a free online tool that displays the solutions for the given expression using the distributive property. BYJU’S online distributive property calculator tool makes the calculations faster and it displays the simplification of . The Distributive Property states that, for real numbers a, b, and c, two conditions are always true: a(b + c) = ab + ac. a(b - c) = ab - ac. You can use distributive property to turn one complex multiplication equation into two simpler multiplication problems, then add or subtract the two answers as required. Distributive property of multiplication
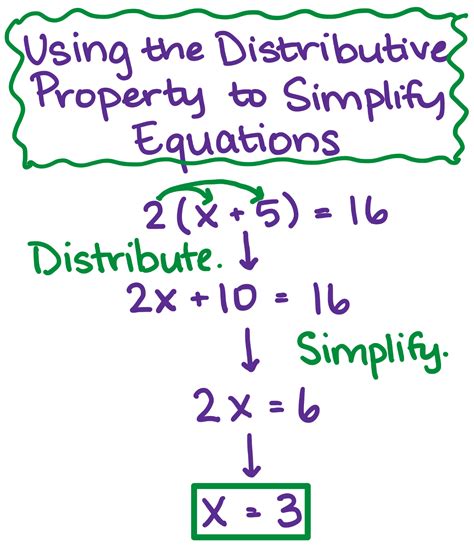
The Distributive Property states that if a, b, c are real numbers, then a(b + c) = ab + ac. In algebra, we use the Distributive Property to remove parentheses as we simplify expressions. When . The distributive property refers to the distributive property of multiplication and applies to both addition and subtraction. An expression in the form A × (B + C) is solved as (A × B) + (A × C) using the distributive property. The distributive property is the rule that relates addition and multiplication. Specifically, it states that \( a(b+c) = ab + ac \) \( (a+b)c = ac + bc .\) It is a useful tool for expanding expressions, evaluating expressions, and simplifying expressions.
distributive property subtraction

distributive property formula
distributive property for sale
New and used Tool Boxes for sale in Grand Junction, Colorado on Facebook Marketplace. Find great deals and sell your items for free.
a b x c d distributive property in boxes|how to use distributive property