a uniform piece of sheet metal is shaped There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal . The DOHO electrical enclosure provides a secure, weatherproof shelter for your electrical system while maintaining the ideal temperature to prevent overheating. This NEMA-rated enclosure comes in a variety of sizes and custom options to ensure that you get the right fit for your needs.
0 · Solved A uniform piece of sheet metal is shaped as shown in
1 · SOLVED: A uniform piece of sheet metal is shaped as shown in
2 · Q48P A uniform piece of sheet metal i [FREE SOLUTION]
3 · Center mass of uniform sheet of steel
4 · Answered: A uniform piece of sheet metal is
5 · A uniform piece of sheetmetal is shaped as shown in
6 · A uniform piece of sheet metal is shaped as shown in the
7 · A uniform piece of sheet metal is shaped as shown in
Buy Wago Junction Boxes at Screwfix.com. Buy online & collect in hundreds of stores in as little as 1 minute! Over 40,000 products. Free next day delivery available. Free returns.
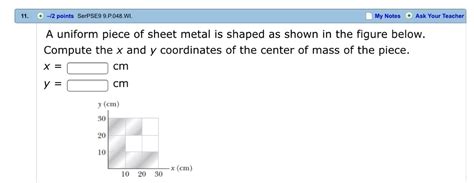
A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.
There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal .A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece .
Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume . First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y.
Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X= cm y.A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.
There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. x = cm y = cm y (cm) 30 20 10 -x (cm) 10 20 30.A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece of sheet metal placed on the first quadrant. The graph is shown to have nine cells, each with a width and height of 10 cm, with three cells on each row. Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)
We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume that the mass is evenly distributed throughout the shape.
First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of the rectangle a, the height of the rectangle b, and the height of the triangle c. The area of the rectangle is A 1 = a b, and the area of the triangle is A 2 = 1 2 a c .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y. Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can be done using the formula x̄ = ∑mx/∑m and ȳ = ∑my/∑m, where m is the mass of each element and x and y are the coordinates of the element.
Solved A uniform piece of sheet metal is shaped as shown in
Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X= cm y.A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.
There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. x = cm y = cm y (cm) 30 20 10 -x (cm) 10 20 30.
A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece of sheet metal placed on the first quadrant. The graph is shown to have nine cells, each with a width and height of 10 cm, with three cells on each row. Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)
We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume that the mass is evenly distributed throughout the shape. First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of the rectangle a, the height of the rectangle b, and the height of the triangle c. The area of the rectangle is A 1 = a b, and the area of the triangle is A 2 = 1 2 a c .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y.
Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can be done using the formula x̄ = ∑mx/∑m and ȳ = ∑my/∑m, where m is the mass of each element and x and y are the coordinates of the element.
SOLVED: A uniform piece of sheet metal is shaped as shown in
desktop cnc milling machine aluminium
determining shape of distribution box plot

Q48P A uniform piece of sheet metal i [FREE SOLUTION]
As of July 28, 2017 Waterloo Industries has been acquired by The Stanley Black & Decker Corporation. Please visit one of our brand sites shown below for all your metal storage needs.
a uniform piece of sheet metal is shaped|A uniform piece of sheetmetal is shaped as shown in